Fundamental class

For the fundamental class in class field theory see class formation.

In mathematics, the fundamental class is a homology class [M] associated to an oriented manifold M, which corresponds to "the whole manifold", and pairing with which corresponds to "integrating over the manifold". Intuitively, the fundamental class can be thought of as the sum of the (top-dimensional) simplices of a suitable triangulation of the manifold.

1 Definition
2 Poincaré duality
3 Applications
4 See also

Definition

Closed, orientable

When M is a connected orientable closed manifold of dimension n, the top homology group is infinite cyclic: 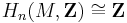$H_n(M,\mathbf{Z}) \cong \mathbf{Z}$ , and an orientation is a choice of generator, a choice of isomorphism 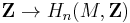$\mathbf{Z} \to H_n(M,\mathbf{Z})$ . The generator is called the fundamental class.

If M is disconnected (but still orientable), a fundamental class is a fundamental class for each connected component (corresponding to an orientation for each component).

It represents, in a sense, integration over M, and in relation with de Rham cohomology it is exactly that; namely for M a smooth manifold, an n-form ω can be paired with the fundamental class as

$\langle\omega, [M]\rangle = \int_M \omega$

to get a real number, which is the integral of ω over M, and depends only on the cohomology class of ω.

Non-orientable

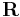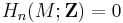If M is not orientable, one cannot define a fundamental class, or more precisely, one cannot define a fundamental class over $\mathbf{Z}$ (or over $\mathbf{R}$ ), as $H_n(M;\mathbf{Z})=0$ (if M is connected), and indeed, one cannot integrate differential n-forms over non-orientable manifolds.

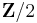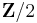However, every closed manifold is $\mathbf{Z}/2$ -orientable, and $H_n(M;\mathbf{Z}/2)=\mathbf{Z}/2$ (for M connected). Thus every closed manifold is $\mathbf{Z}/2$ -oriented (not just orientable: there is no ambiguity in choice of orientation), and has a 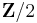$\mathbf{Z}/2$ -fundamental class.

This 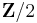$\mathbf{Z}/2$ -fundamental class is used in defining Stiefel–Whitney numbers.

With boundary

If M is a compact orientable manifold with boundary, then the top relative homology group is again infinite cyclic $H_n(M,\partial M)\cong \mathbf{Z}$ , and as with closed manifolds, a choice of isomorphism is a fundamental class.

Poincaré duality

Main article: Poincaré duality

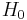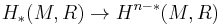Under Poincaré duality, the fundamental class is dual to the bottom class of a connected manifold (a generator of $H_0$ ): in the closed case, Poincaré duality is the statement that the cap product with the fundamental class yields an isomorphism $H_* (M, R) \to H^{n-*}(M, R)$ .

Applications

In the Bruhat decomposition of the flag variety of a Lie group, the fundamental class corresponds to the top-dimension Schubert cell, or equivalently the longest element of a Coxeter group.